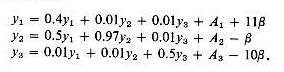
International Economics, Robert A. Mundell, New York: Macmillan, 1968, pp. 100-107.
The Laws of Comparative Statics and
Homogeneity1
|
It is well known that there are formal structural similarities between economic models that encompass quite different subject matters. We cite, for example, the similarities between the Walrasian multiple-commodity price system and the Metzleric multiple-region income system.2
These similarities produce analogies between the laws of stability and comparative statics of the two systems that further our understanding of the basic unity of economic science. The analogies are produced by an underlying mathematical isomorphism that is, however, not complete.
The mathematical isomorphism between the Walrasian multi-commodity price system and the Metzleric multi-region income system is not complete even in the well-behaved case of gross substitutes (for the stable price system) and superior goods (for the stable income system). A difference arises because of the price system's characteristic of zero-degree homogeneity that imposes additional constraints upon the inverse. The inverse of the price system has diagonal elements in both the same row and the same column, while the inverse of the income system has diagonal elements that are necessarily dominant only over single elements in the same column.
This leads to a whole class of comparative statics propositions for the price system that have no counterpart in the income system, and to economic implications about our ability to aggregate sectors or markets for qualitative analysis.
Consider a Walrasian system in which all goods are gross substitutes, and a Metzleric system in which all income effects (including hoarding) are positive; then both systems are stable.3 Now consider three types of changes in (excess) demand and note the laws of comparative statics (proved in the final section) pertaining to each system. In the Walrasian system it can be demonstrated4 that:
I. A shift of demand from the numéraire to good k (a) raises the price of k and the price of all other goods and (b) raises the price of all other goods by less than the price of k.
II. A shift of demand from good i to good k (a) raises the price of k and lowers the price of i and (b) lowers the price of every other good by less than the price of i or raises it by less than the price of k.
III. A shift of demand from all other goods onto good k (a) raises the price of k and (b) lowers the price of all other goods in terms of k.
On the other hand, in the Metzleric system, it can only be proved5 that:
I. A shift of demand from saving onto the goods of country k raises the income of country k and raises income in every other country; it cannot be proved that income in any other country rises by less than income in country k.
II. A shift of demand from country i to country k raises income in country k and lowers income in country i; it cannot be proved that incomes in other countries rise by less than in country k or fall by less than in country i.
III. A shift of demand from all other countries onto country k raises income in country k; it cannot be demonstrated that incomes in other countries fall, or rise by less than income in country k (although income in at least one other country must fall).
It can be seen that there are analogies in the Metzleric system to propositions I(a), II(a), and III(a) of the Walrasian system, but there are no analogies to propositions I(b), II(b), and III(b). Those propositions in the Walrasian system that have no counterpart in the Metzleric system all have to do with changes in the price of one good expressed in terms of a nonnuméraire good; we cannot assert much about the differences between income increases in various countries. The economic explanation is that we cannot apply the additional information that we have available in the Walrasian system, that is, that the system is homogeneous of degree zero, to the Metzleric system. The (a) propositions in the Walrasian system do not depend on homogeneity, but the (b) propositions require additional information such as that provided by, homogeneity.
Most propositions of comparative statics in economic theory have been derived originally from simple models involving only two or three entities (goods, factors, regions, countries), although such propositions are useful only if there is at least one general case in which the same propositions are applicable. The usefulness of the gross substitutes case of the Walrasian: system lies partially in its affirmation of the propositions derived from simple two-good systems: If all goods are gross substitutes, one good can be taken to represent the remaining goods, and qualitative analysis can be much simplified. By analogy it might appear that the " normal case " of the multiple-region income system would have a similar utility. Thus Metzler ([64], p. 352) has concluded, for the multiple-region income model, that there "are no processes of income adjustment in the n-country model that are not also revealed by the simple two-country model, and in the main the conclusions reached by employing the latter are the same as those reached by employing the former."
Given, however, the dissimilarity between the comparative statics propositions pertaining to the two systems, and (specifically) the additional information provided by the homogeneity postulate in the Walrasian system,: it seems unlikely that the similarity of results between two-entity and multiple-entity systems is not influenced by the homogeneity postulate. In other words, is it not possible to find some discrepancies between the conclusions of two-region and multiple-region income models that are based on the absence of homogeneity ?
Let us consider a two-region model with one region representing the rest
of the world. Suppose we try to determine the effects that a shift in demand;
from the rest of the world onto the products of one country would have on
the income in that country and in the rest of the world. This involves (by
assumption) no change in aggregate spending in the world as a whole, so world
saving remains constant. It should be apparent then that income in the country
for which demand has increased must rise while income in the country for
which demand has decreased must fall, since only in that way can world saving
remain constant. In other words we should conclude, on the basis of the
two-region model with one region representing the rest of the world, that
a shift of demand from the rest of the world onto one region raises income
in that region and lowers income in the rest of the world.
Consider, however, the following example of a three-country world, where
y represents income in the country denoted by the subscript, beta
is a parameter, and the A's are constants:
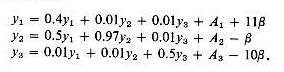
This is a " normal " Metzleric model. The coefficients of the parameter beta specify that aggregate world demand (at constant incomes) is unchanged by a shift in the parameter. Yet the approximate solutions for the changes in incomes of the three countries due to a change in beta are dy1/dbeta = 24.2, dy2/dbeta = 366.6, and dy3/dbeta = -12.2. This shows that a shift of demand onto one country from the rest of the world can raise income in the world as a whole and can also raise income in that part of the world for which demand has initially decreased.
My example does not refute Metzler's statement, but it does prove that it must be carefully interpreted. There is, in fact, an analogy in the multiple-region system that corresponds to that implied by the two-region system. When it is proved, in the two-region income model, that income in one region falls and income in the other region rises, the interpretation must be that a shift of demand from one region onto another region raises income in the latter region and lowers income in the former region. The error in the previous interpretation is that one region was taken to represent the rest of the world, a representation that is generally invalid in a nonhomogeneous system.6
Let us now prove the laws of comparative statics advanced in the first section for the Walrasian and Metzleric systems. Consider the Walrasian system with n + 1 goods:
![]()
where Bi is the excess demand for the ith good, the p's are prices expressed in terms of the numéraire (good 0), and | alpha | is a parameter; compare (1) with the n-country Metzleric system:
![]()
where Xi is the aggregate excess demand for the goods of the ith country, the y's are national incomes expressed in a common currency unit, and beta is a parameter.
Choose commodity units in (1) so that initially each pi = 1, and differentiate (1) and (2) with respect to the parameters alpha and beta. The solutions are

with
![]() ,
and where Dij is the cofactor of the
ith row and jth column of D. M is the Metzleric
determinant.
,
and where Dij is the cofactor of the
ith row and jth column of D. M is the Metzleric
determinant.

and Mij is the cofactor of the ith row and ith column of M, while c represents the marginal propensity to consume home goods in the country denoted by the subscript, and mij denotes the marginal propensity in j to import from i.
Determinants D and M have, by assumption, positive off-diagonal elements and negative column sums. This yields two properties in common:
1. Every first-order cofactor has a sign opposite that of the basic determinant,7 that is, Dij/D < O, and Mji/M < O.
2. In absolute value every principal cofactor dominates off-diagonal cofactors in the same column as the principal cofactor,8 that is, | Dii | , > | Dji | and | Mii | > | Mji |.
Homogeneity, however, provides additional information about D. If the system (1) is homogeneous in all n + 1 prices, then the row sums must also be negative, and this implies that principal cofactors in D will dominate off-diagonal cofactors in the same row as the principal cofactor. An implication of homogeneity is therefore that | Dii | > | Dij | 9; an analogous result, | Mii | > | Mij |, cannot be asserted for the Metzleric system.l0
These properties can now be utilized to prove propositions I, II, and III. To prove the first proposition set deltaBj/delta alpha = 1 and every other deltaBj/delta alpha = 0; and set deltaXk/delta beta = 1 and every other deltaXj//delta beta = 0. Then from equations (3) we have

which proves proposition I(a) using Mosak's theorem; and

But the sign of
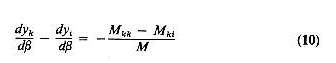
is indeterminate for any particular i because we do not have the
information provided by homogeneity.
To prove proposition II(a) we can set -
deltaBi /delta alpha =
deltaBk/delta alpha = 1 in the Walrasian system
and drop all other coefficients; and set -
deltaXi /delta beta =
deltaXk/delta beta = 1 in the Metzleric system and drop all
other coefficients. Then we have, from (3),

These results do not depend on homogeneity.
To prove proposition III and also, indirectly, proposition II(b), set

in (4). Then, after eliminating delta
Bk/delta alpha from (3) we have

This proves proposition III(a).
To prove proposition III(b) we need to prove that
dpk/d alpha -
dpi/d alpha > 0. Solving for delta
Bk/delta alpha from (3) and eliminating it
from the expression for dpk/d alpha - dpi/d
alpha we find that

Now the numerator of the last expression on the right in (17) is positive by Mosak's theorem and the stability proofs since, for any determinant D,
DDkk,ji = DjiDkk - DjkDki ;
and the first expression on the right of (17) is positive, by the homogeneity postulate and by (15). Therefore, both terms on the right of (17) are positive, so the price of the good for which demand has increased rises relative to all other prices. A similar proof can be applied to prove II(b) and to demonstrate that the expression on the right of (11) exceeds the expression for the change in any other price, and that the expression on the right of (12) is less than the X expression for any other price change.
We cannot apply the same method to prove a theorem analogous to propositions
II(b) or III(b) for the income theory, because we would always have an expression
like | Mkk | - |
Mki |, whose sign is indeterminate. Nor
can analogous theorems exist, as the counterexample in this chapter illustrates.
We can, however, demonstrate that incomes in one of the countries for which
demand has decreased must fall in case III. Because world expenditure is
unchanged (at constant incomes), world saving must be unchanged,
so
![]() . Thus, if income
in one country increases, income in at , least one other country must decrease.
. Thus, if income
in one country increases, income in at , least one other country must decrease.
______________________________
Notes
1 Adapted from Econometrica, 33, No. 2 (April 1965), pp. 349-356. Original title: "The Homogeneity Postulate and the Laws of Comparative Statics."
2 For a description of the
"Walrasian" system see, for example Hicks ([24], chap. V) Samuelson ([91],
pp. 269-276), or Metzler [62]- for the "Metzleric" system see Metzler [64],
Chipman [7], or Goodwin [17]. Metzler's paper, although published in 1950,
was written in 1945.
3 This follows because the
diagonal elements of the characteristic matrix are in each case dominant.
For proofs for the Metzleric system see [7], [17], and [64]; for the Walrasian
system see [1], [19], and [79]. Relevant mathematical generalizations have
been given by Solow [98], Debreu and Herstein [10], and Mackenzie [49] for
indecomposable matrices, nonnegative square matrices, and dominant-diagonal
matrices, respectively. The mathematical contribution of this chapter is
to show that it is useful to distinguish between "one-way" and "two-way"
dominance of the diagonals in the basic matrices for comparative statics
purposes; see the last section.
4 Class I propositions are well known from Hicks ([24], p. 75); Morishima [67] proves their validity for finite shifts in demand. Class II propositions have not, to my knowledge, been proved before, although any economist's intuition must tell him they are correct if class I propositions hold, since they differ only in the choice of numéraire. Class III propositions have been used in my papers [70 and 74]. See chapters 3 and 4.
5 Class I and class II
propositions of the Metzleric system have been proved by Metzler in [64
and 65], respectively. Class III propositions are proved in the final section.
6 Perhaps the most obvious
economic implication of the possibilities we have raised is that devaluation
and tariffs are not necessarily beggar-thy-neighbor policies even when the
entire world is in a state of unemployment, at least one neighbor gets "
beggared," but the combined income of the rest of the world may rise.
7 By Mosak's theorem ([68], pp. 49-51).
Its application to the income system is due to Metzler ([64], P. 340).
8 See Metzler ([65], p. 21).
9 This follows by analogy to Metzler's
result. For example, in the 2 X 2 case the basic determinant is
![]()
has a sign opposite to D.
10 In a two-region model the basic
determinant is
![]()
and there is no economic reason why its sign should be determinate.
____________________________________
[1] K. J. ARROW and L. HURWICZ, "On the Stability of the Competitive Equilibrium, I," Econometrica, 26, 522-552 (Oct. 1958).
[7] J. S. Chipman, "The Multi-Sector Multiplier," Econometrica, 18, 355-374 (Oct. 1950).
[10] G. DEBREU and I. N. HERSTEIN, " Non-negative Square Matrices,' Econometrica, 21, 597-607 (1953).
[11] DE NEDERLANDSCHE BANK N.V., Report of the Year 1960. Amsterdam: 1961.
[17] R. M. GOODWIN, "The Multiplier as Matrix," Econ. Jour., 59, 537-555 (Dec. 1949).
[19] F. HAHN, "Gross Substitutes and the Dynamic Stability of General Equilibrium," Econometrica, 26, 169-170 (Jan. 1958).
[24] J. R. HICKS, Value and Capital. 2nd ed. Fair Lawn, N.J.: Oxford University Press, 1946.
[62] L. A. METZLER, "Stability of Multiple Markets: The Hicks Conditions," Econometrica, 13, 277-292 (Oct. 1945).
[64] L. A. METZLER, "A Multiple-Region Theory of Income and Trade," Econometrica, 18, 329-354 (Oct. 1950).
[65] L. A. METZLER, "A Multiple-Country Theory of Income Transfers," Jour. Pol. Econ., 59, 14 29 (Feb. 1951).
[67] M. MORISHIMA, " On the Three Hicksian Laws of Comparative Statics," Rev. Econ. Stud., 27, 195-205 (1959-1960).
[68] J. L. MOSAK, General Equilibrium Theory in International Trade. Bloomington: Indiana University Press, 1944.
[70] R. A. MUNDELL, "The Pure Theory of International Trade," Amer. Econ. Rev., 50, 67-110 (March 1960).
[74] R. A. MUNDELL, "Tariff Preferences and the Terms of Trade," Man chester School Econ. Soc. Stud., 32, 1-13 (Jan. 1964).
[79] T. NEGISHI, "A Note on the Stability of an Economy where A11 Goods Are Gross Substitutes," Econometrica, 26, 445-447 (July 1958).
[91] P. A. SAMUELSON, Foundations of Economic Analysis. Cambridge: Harvard University Press, 1953.
[98] R. SOLOW, "On the Structure of Linear Models," Econometrica, 20, 29-46 (Jan.1952).